I've been continuing to work my way through Barry Luokkala'a great book, Exploring Science through Science Fiction - and I really liked his take on this scene, where Spiderman stops a runaway train by attaching webs to the buildings nearby. I set it as a challenge to a Leaving Cert class yesterday and they seemed to enjoy it, while also revising the equations of motion, kinetic energy, work, and Hooke's Law...
The philosophical contortions required to take this seriously are part of the fun: we have to accept the whole idea that you can be turned into a spider-like superhero by being bitten by a radioactive spider and that you are therefore capable of creating vast web-like structures with minimal effort, and then we can focus on the physics of one small aspect of this scene - could a spiders webs be strong enough to atop a train, if they were big enough.
To answer the question, you have to simplify it and what he does is to treat a web like a spring, which seems fair enough, and to calculate the Spring constant (k) . If this is in keeping with the value of k for actual spider's webs (when suitably scaled up) then the scene is plausible. And to find a value for k, he makes a few assumptions based on the evidence available in the scene: a NY transit train typically has a mass of 155,000 kg, it is travelling at 88 mph (which I rounded to 140 km/hr) and it is stopped over a period of 50 seconds. I had to adapt the calculations that followed to fit them in with Leaving Cert Physics, but that was easy enough.
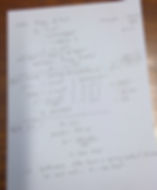
Apparently the strength of a web is best measured using Young's Modulus (Y). Which relates to the spring constant but also to the length and diameter of the web - and that's why we can always pull a web apart: the diameter is so small, and we're so big, that its overall strength doesn't seem so impressive to us. But if you could scale a web up to something with a diameter even in the centimetre range, you would have a phenomenally strong material.
But rather than introducing a whole new concept to Leaving Cert students, I just adapted the range of values for Y, to get the equivalent values of k. And apparently, if scaled up, spiders webs would have values for k ranging from 100 N/m up to 25,000 N/m.
So our value above is actually quite low for a web. And therefore the scene is plausible! (As long as you accept that you can be turned into a spider-like superhero by being bitten by a radioactive spider and that you are therefore capable of creating vast web-like structures with minimal effort)
I worked it all into a PowerPoint to work it through with a class, and I've uploaded it here, if anybody is interested....